Angle of Impact Calculator

Learn one of the fundamentals of forensics with our angle of impact calculator: here, helped by a bit of math, you'll discover how detectives recreate one of the elements of a crime scene.

Here we will explain:

• What is the angle of impact;
• How to calculate the angle of impact;
• Where we use the angle of impact; and
• Forensics at home.

Don your gloves and follow the clues with Omni Calculator.

Trials and procedural justice lacked a solid and reliable scientific base for a long time. The best source of information was usually — and in the best case — a witness. More often than not, this resulted in unfair, wrong, or downright malicious outcomes of criminal procedures.

Then, around the time of the Enlightenment science and reason came into the spotlight. The justice system started introducing elements of pathology, physiology, chemistry, and physics in court, alongside "simple" human witnesses.

In an empowering move, numbers and facts made trials fairer. From then on, it was only going to get better: starting from simple anatomical analysis, we ended a few centuries later with a complex and refined set of tools spanning from DNA analysis to computerized tools that allow building a crime scene back from latent elements.

In case the victim suffered blood loss, droplets are one of the most valuable tools in the shed of criminologists, helping them reconstruct the dynamics of the crime.

Crime scenes are often bloody: forensic scientists managed to turn murder into number and developed a framework able to roughly understand the dynamics of a crime through the analysis of the shape of blood droplets.

Blood droplets travel with an almost spherical shape, and then... splat! Droplets land, creating a specific elongated shape (an ellipse), containing information on the angle at which they impact the final surface.

The calculations of the angle of impact of blood spatter followed — and complete — the analysis of the horizontal direction of the droplets. This first step is pretty straightforward: the splatter's elongated shape suggests to the detectives the direction from which the droplets came.

Tracing a set of lines along the major axis of the ellipses of each blood droplet converges in the surrounding of a point: that's the location of the "spill". If "major axis" sounds mysterious to you, visit the ellipse calculator for a quick refresh!

Anyway, back to the problem. What do we know about the height of the wound?

The location of a wound tells a story: was the victim lying down, sitting, or standing? Maybe even suspended. The height at which a blood drop starts its quick journey to the floor contains crucial information that often helps in the resolution of a case.

That information is encoded in the angle of impact of blood spatter: we define this quantity as the angle between the impact surface and the trajectory of the splatter itself. The angle of impact can vary from $0\degree$ (excluded) to $90\degree$ (included). Its value dramatically affects the final shape of blood spatters: look at the figure below!

As you can see, an angle of impact equal to $90\degree$ corresponds to a (theoretically) circular shape: the blood dripped and was not traveling far from the body.

The lower the angle, the more elongated the shape becomes: eventually, the angle is so low that the shape is only a long smear on the surface.

Together with assessing the horizontal provenience of the droplets, the calculation of the angle of impact composes the broader bloodstain pattern analysis. Let's proceed with our investigation with the formula for the angle of impact of a blood droplet.

Given the great importance of blood pattern analysis, you'll be pleasantly surprised by the innocence of the formula than tells you how to calculate the angle of impact of a blood spatter.

Once you reach the crime scene, you need to whip out a ruler and start measuring the shape of each blood spatter: we are interested in two quantities:

• The major axis $L$; and
• The minor axis $W$.

The two elements are straightforward to identify in the typical elliptical shape of the final form of a blood droplet.

The bloodstain may have a tail, an irregular ending that smears the elliptic shape. Don't worry about it, and ignore it in your measurements: this won't be the last approximation leading to the formula for the angle of impact.

Your colleague, detective Perry Masin is here to help. The sine of the angle of impact equals the ratio between the two axes of the ellipse; to calculate the angle itself, we make use of the arcsine function: an inverse trigonometric function (you can learn more about it at our arcsin calculator).

We told you that the expression was simple!

Now, to some sober reality: the calculations for the angle of impact are almost criminally approximated. Many factors, from gravity (we assume that the blood drops travel in a straight line, point-blank, and not following a parabola as we saw in the trajectory calculator) to blood viscosity or air resistance, don't enter the formula. It's hard to quantify them, and — at least in part — they probably cancel each other contributions. Still, those approximations affect the final result, introducing errors as big as $50\%$ of the actual value.

We now know that the analysis of the angle of impact is approximate. Is it still helpful? Yes!

After calculating angles of impact and horizontal direction, forensic scientists start drawing lines, both:

• Following the horizontal direction departing from the center of the stain; and
• Rising from the surfaces with the value calculated with the formula for the angle of impact of a blood droplet.

Eventually, those lines more or less converge in a particular area, called area of convergence appropriately. We can infer that the blood originated from that area.

Professionals call this process stringing: it became popular due to the TV show Dexter, but it has comprehensive and valuable use, unlike many other fictional forensic techniques shown on the screen!

You are on the crime scene, and you can see blood spatters everywhere around you. Let's see if we can reconstruct what happened here.

Take three splatters, and measure them. You found:

• $W_1 = 2.1\ \text{cm}$ and $L_1=2.3\ \text{cm}$ for the first one;
• $W_2 = 1.4\ \text{cm}$ and $L_2 =1.7\ \text{cm}$ for the second one; and
• $W_3 =1.9\ \text{cm}$ and $L_3= 3.1\ \text{cm}$ for the third one.

The horizontal analysis suggests that the blood droplets originated from a distance of, respectively:

• $33\ \text{cm}$ (first droplet);
• $52\ \text{cm}$(second droplet); and
• $98\ \text{cm}$ (third droplet).

Now we have enough data to start looking for an answer. Take the formula for the angle of impact, and feed it the first splatter's measurements:

Considering the angle and the distance, we can infer that the point of origin lies at about $74\ \text{cm}$ from the ground (we used the trigonometric equality $33\tan{(65.93\degree)}=73.91\simeq74$ for a right triangle: learn how at the right triangle side and angle calculator).

To seek confirmation, let's calculate the angle of impact and then the height of the other droplets. Use our calculator to find the values: insert the width and length of the spatter, using the correct measurement units.

For the angle of impact, we find:

Corresponding to the height calculated with the tangent of these angles, respectively of $75\ \text{cm}$ and $76\ \text{cm}$.

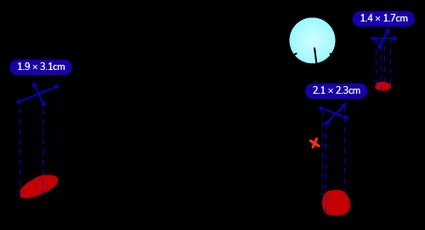
As you can see, the results of this "mathematical" stringing lead us to a spot a few centimeters wide, about $75\ \text{cm}$ high. The victim was not standing, nor were they on the floor. Maybe kneeling?

You can experiment with the angle of impact at your home. Prepare a fluid with a behavior similar to the one of blood, and try to throw some drops on a floor, measure them and calculate the angle of impact!

Here's a recipe for fake blood. Feel free to modify it until it feels "right". Mix:

• 4-5 tablespoons of corn starch;
• 1 gallon of water.

We skipped the coloring because it would stain a lot! Boil the concoction (slowly) and let it simmer for a few minutes. Wait for it to cool down, and eventually add some dish soap to give it a better texture.

Now, start dripping and measuring. Then check if the science works!

You learned that you could reconstruct a small part of a crime scene with a bit of math and a ruler. Isn't it amazing? However, keep in mind the many simplifications introduced to make this forensic tool easy to use. We hadn't been scared of approximating; from ignoring the viscosity to assuming that the droplets travel in a straight line, we hadn't been scared of approximating!

We are glad we could be your accomplices in this short introduction to forensic science. And trust us, our angle of impact calculator is a truthful witness. The case is closed!