Square Calc: find A, P, d
With this simple square calculator, you can find all the parameters in the blink of an eye. Fill in one box, and the calculator will display all the remaining parameters: side, area, perimeter, and diagonal. Scroll down if you want to remind yourself about the basic formulas in square calculations.
What is a square?
A square is a quadrilateral (a shape we met in our quadrilateral area calculator) with four equal sides and four equal angles (right angles = ). It's one of the most basic shapes.
It's a special case of a rhombus, a kite, a trapezoid, a parallelogram, and a rectangle, so it has all properties of these shapes.
Square calc: find P (perimeter)
The perimeter of a square is equal to the sum of all sides. Because the square has all four sides equal, the perimeter is then:
Square calc: find A (area)
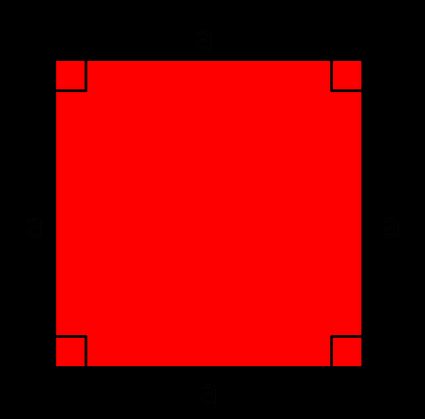
The area of a square is the product of the length of its sides:
where is a square side
The term square is used to describe multiplying the value by itself, raising it to the second power.
Squares are, by large, the easiest shape when it comes to calculate the area. For other quadrilaterals, formulas may get complex, as you can see in our area of a trapezoid calculator: however, even if there are many types of trapezoids, one formula fits them all. You can also meet the generalization of the formulas above in our area of a rectangle calculator, and discover what happens when you deform your rectangle at our parallelogram area calculator.
Square calc: find d (diagonal)

Diagonals of a square are equal in length, they bisect the angles, and they are the perpendicular bisectors of each other. The diagonal of a square is equal to the side length times the square root of :
So, for example, if the square side is equal to , then the diagonal is .
Square properties
The Square is a unique shape. Here we sum up a few important features:
- Equality: all four angles are equal, all four sides of a square are equal, and both diagonals are of the same length.
- Bisection: diagonals bisect each other and bisect square angles.
- Perpendicularity: all square angles are equal to and the diagonals meet at .
How to use square calc: find perimeter example
Now, as you read through the formulas and you know precisely what the square is, let's have a look at this simple example:
Assume that you want to calculate the perimeter, area, and diagonal of your square table. The only thing given is side length - .
- To calculate the area, multiply the side length by itself. It'll give us .
- To determine the perimeter, multiply the side length by . In our case, the result is .
- To determine the diagonals, multiply (about ) times the length of a side. It's .
- Or simply use our square calc to find the diagonals, area, and perimeter of a square.
