Arctan Calculator (Inverse Tangent)

Use this arctan calculator to quickly find the inverse tangent. Whether you're looking for a simple answer to the question "what is an arctan?" or are curious about the integral or derivative of arctan, you've come to the right place. Below, you'll also find the arctan graph, as well as a neat table with commonly used values, such as arctan(1) and arctan(0). Alternatively, you can simply type the value of interest into this tool, and you'll find the answer in the blink of an eye.

Interested in more advanced trigonometry? Check out our law of sines calculator and law of cosines calculator calculators if you have any triangles to solve.

Arctangent is the inverse of the tangent function. For an in-depth analysis of the tangent, visit our tangent calculator. Simply speaking, we use arctan when we want to find an angle for which we know the tangent value.

However, in the strictest sense, because the tangent is a periodic trigonometric function, it doesn't have an inverse function. Still, we can define an inverse function if we restrict the domain to the interval where the function is monotonic. The commonly chosen interval, $-\frac{\pi}{2}<y<\frac{\pi}{2}$, is called a principal value and is, at the same time, the range of an inverse tangent function.

Using the $\tan^{-1}(x)$ convention may lead to confusion about the difference between arctangent and cotangent. It turns out that arctan and cot are really separate things:

• $\cot(x) = 1/\tan(x)$, so cotangent is basically the reciprocal of a tangent, or, in other words, the multiplicative inverse.
• $\arctan(x)$ is the angle whose tangent is $x$.

We hope that now you do not doubt that arctan and cotan are different. To avoid any further misunderstandings, you may want to use the $\arctan(x)$ rather than $\tan^{-1}(x)$ notation.

By restricting the domain of the principal tangent function, we obtain the inverse tangent that ranges from $-\frac{\pi}{2}<y<\frac{\pi}{2}$ radians exclusively. However, the domain of an arctangent function is all real numbers. The graph then looks as follows:

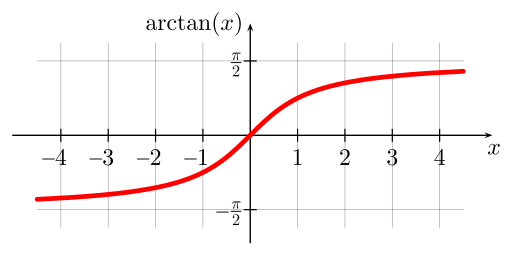
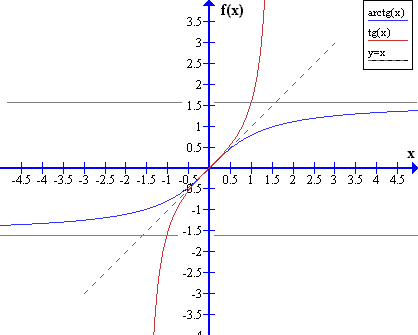
How is this arctan graph created? By reflecting the $\tan(x)$ in the $(-\pi/2, \pi/2)$ range through the line $y = x$. You can also look at it as swapping the horizontal and vertical axes:

The relationships in trigonometry are crucial to understanding this topic even more thoroughly. Inspecting the right-angled triangle with side lengths $1$ and $x$ is a good starting point if you want to find the relationships between arctan and the basic trigonometric functions:

• Sine: $\sin(\arctan(x)) = x / \sqrt{1 + x^2}$;
• Cosine: $\cos(\arctan(x)) = 1 / \sqrt{1 + x^2}$; and
• Tangent: $\tan(\arctan(x)) = x$.

Other useful relationships with arctangent are:

• $\arctan(x) = \pi/2 -\text{arccot}(x)$.
• $\arctan(-x) = -\arctan(x)$.
• $\arcsin(x) = \arctan\left(x / \sqrt{1 - x^2}\right)$ (meet the arcsin at the arcsin calculator!).
• Integral of arctan:

• Derivative of arctan:

• $\arctan(x) + \arctan(1/x) = \pi/2$, for $x > 0$ and $\arctan(x) + \arctan(1/x) = -\pi/2$, for $x < 0$

It's easy to prove the first equation from the properties of the right triangle with side lengths $1$ and $x$, as we perfectly know that the sum of angles in a triangle equals $180\degree$. Subtracting the right angle, which is $90\degree$, we're left with two non-right angles, which must sum up to $90\degree$. Thus, we can write the angles as $\arctan(x)$ and $\arctan(1/x)$.

This is one of our easiest calculators to use, really! Just enter the number which you want to find the arctan of. As the domain of arctan is all real numbers, you don't need to worry too much. Let's say we want to find the arctan of 1. Just type in the number and the inverse tangent calculator will display the result. As we expected, the arctan of 1 is equal to $45\degree$. This arctan calculator works the other way round as well, that is as a standard tangent calculator - type the angle into the second box and tangent of that angle will appear.

The arctan(x) is equal to the inverse tangent function: tan⁻¹(x). If in a right triangle, the tan of the angle determines the ratio of the perpendicular to the base (tan(x) = perpendicular / base), then arctan will help us find the value of the angle x: x = tan⁻¹(perpendicular / base).

Yes, arctan is the same as tan⁻¹ and means the inverse tangent function. However, be careful not to confuse tan⁻¹(x) with (tan(x))⁻¹, which means 1/tan(x) and refers to other trigonometric function, the cotangent of x.

Arctan 1 (or tan inverse 1) gives the angle measure of a right-angled triangle in which the ratio of the opposite side and the adjacent side to the angle is equal to 1. The value of arctan 1 is 45° or π/4 radians.

The exact value of arctan(√3) is 60° or π/3. Since tan(60°) = √3 and arctan is the inverse tan function, arctan(√3) will be 60°.

To draw the graph of arctan and read values for certain angles:

1. Draw a coordinate system, where the y-axis is the horizontal axis, and the x-axis is the vertical axis.
2. Draw the graph of the x = tan(y) but only in the range <-π/2;π/2>.
3. Rotate the graph 90 degrees clockwise.
4. You got it! It's a graph of y = arctan(x).

Since arctan(x) = tan⁻¹(x), the integral of arctan is the integration of the tan inverse x, which we can write mathematically as:

∫tan⁻¹(x) dx = x tan⁻¹(x) − ½ ln |1+x²| + C

In this equation, C is the integration constant, dx denotes that the integration of the tan inverse x is with respect to x, and ∫ denotes the integration symbol.

The derivative of the arctan function is: d/dx(arctan(x)) = 1/(1+x²). The arctan function can be differentiated because its derivative exists at every point of its domain. Looking at the graph of the single period of the function arctan(x), you will notice that the curve is continuous.