Shear Stress Calculator
This shear stress calculator calculates the shear stress due to transverse loads and the shear stress due to torsion (torque) applied on a circular shaft.
The shear stress from transverse forces is critical in the design of thin-walled members. For non-tin-walled members subjected to transverse loads, the most important is usually the axial stress caused by the bending moment. Our stress calculator can also be helpful if you're dealing with axial stress. If that's not the case, use this calculator!
The shear stress equation to use will depend on whether we apply a transverse load to a beam or a torsion couple to a circular shaft. In the following sections, you can look at the formulas for shear stress under these two conditions. We'll show how to calculate the maximum shear stress for many beams and how to calculate the stress at any point.
Transverse shear stress formula
By definition, shear stress is force acting tangent to an area. In a beam of cross-sectional area subjected to a shear force (), the average shear stress () is:
This is just an average, as the shear stress across a beam subjected to transverse shear varies widely, and we usually need to know its maximum value instead of just the average.
You can find the exact shear stress at any vertical distance from the neutral axis with the following equation:
where:
- – Shear stress at a specific distance from the neutral axis;
- – Internal shear force at the section of interest in the beam;
- – First moment (about the neutral axis) of the cross-sectional area that lies above the point where we'll calculate the stress;
- – Section width at the point where we'll calculate the shear stress; and
- – Moment of inertia of the entire cross-sectional area, about the neutral axis (you can find it with our moment of inertia calculator).
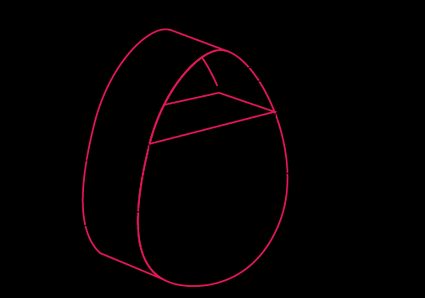
is a tricky quantity. Mathematically, it is the area above the point of interest multiplied by the distance between the centroid of that area to the neutral axis. For example, for the cross-section of cam geometry below, if you wanted to calculate the shear stress at a distance from the neutral axis, the corresponding would be .
Using the shear formula () requires being careful about calculating the different quantities (especially ) and carefully inspecting the point of maximum shear stress (). For that reason, in this calculator, we included the possibility of obtaining for the most common shapes (rectangular, circular, and I-beams) by only inputting their dimensions and the shear force. You can look at those formulas in the table below (scroll to the right to look at them):
Form | Maximum shear stress | |
|---|---|---|
Rectangle | 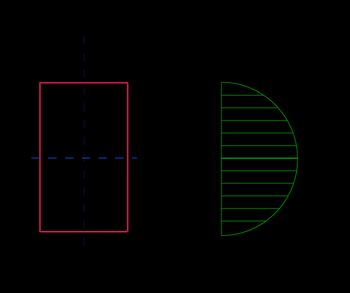 | |
Stress at distance y: | ||
Hollow circle | 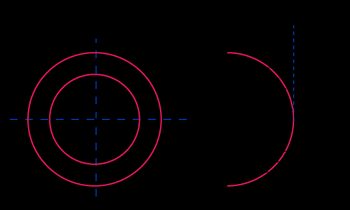 | |
I-beam |  | |
💡 In an I-beam (wide-flange beam), the value (the stress value at the joint between the flange and web) is a critical quantity, as sometimes the joint in that zone is created through welding or glue, and we need to calculate how much stress those elements will withstand.
Torsional shear stress equation - Shear stress due to torsion
To calculate the shear stress from a torque applied to a circular shaft, we use the torsional shear stress formula:
where:
- – Shear stress at the point of interest;
- – Radial distance from the shaft center to the point where we want to calculate the stress (the point of interest);
- – Torque applied to the shaft; and
- – Polar moment of inertia of the cross-section.
- For a solid shaft of radius , .
- For a hollow shaft of inner radius and outer radius , .
The maximum shear stress for torsional loads occurs at the outer surface of the circular shaft, where . In other words, the maximum shear stress () is given by:
Important considerations when using this calculator and the shear stress equations
Please keep this in mind:
- When using these formulas (and most of the formulas in mechanics of materials), you must consider that they apply to homogenous materials with a linear elastic behavior.
- When we apply a transverse load to a beam, transverse and longitudinal shear stresses arise. Due to the complementary property of shear, these longitudinal and transversal stresses have the same magnitude. Therefore, the horizontal shear stress calculation only requires knowing the transverse shear stress value, as they are both the same.
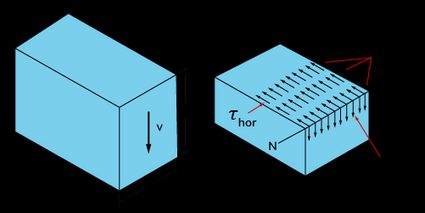
- The shear stress not only varies vertically about the neutral axis but also across the width of the beam. Therefore, the shear stress equation and its derivatives also give us a (horizontal) average stress value (). For "short" or "flat" cross-sections, the deviation of the real stress from is high. For example, the maximum and minimum shear stress in rectangular beams (at its center) increase their deviation from as the base-to-height ratio () increases:
0.25 | 0.5 | 1 | 2 | 4 | 6 | 10 | 20 | 50 | |
|---|---|---|---|---|---|---|---|---|---|
1.008 | 1.033 | 1.126 | 1.396 | 1.988 | 2.582 | 3.770 | 6.740 | 15.65 | |
0.996 | 0.983 | 0.940 | 0.856 | 0.805 | 0.800 | 0.800 | 0.800 | 0.800 |
FAQs
What are the shear stress units?
The shear stress units are pascal (Pa) in the International System of Units and pound per square inch (psi) in the United States customary units system. As stress is usually a big number, other used shear stress units are kPa, MPa, or kpsi.
What is the polar moment of inertia of a circle of 10 cm radius?
The polar moment of inertia of a circle of 10 cm radius is 15708 cm⁴. In the United States customary units system units, the polar moment of inertia is 377.4 in⁴.
How do I calculate the shear stress of a fluid?
To calculate the shear stress 𝜏yx of a fluid:
- Determine whether it is a Newtonian or non-Newtonian fluid.
- For a Newtonian fluid, multiply the viscosity μ by the shear rate du/dy: 𝜏yx = μ(du/dy).
- If it's non-Newtonian, multiply the apparent viscosity η by the shear rate du/dy:
𝜏yx = η(du/dy).
η and μ are analog terms, with the difference that η varies with du/dy.
How do I calculate viscosity from shear stress and shear rate?
To calculate viscosity μ from shear stress 𝜏yx and shear rate du/dy, divide the shear stress by the shear strain:
μ = 𝜏yx/(du/dy)
How do I calculate shear stress in bolts?
The maximum shear stress of a screw body subjected to torsion is:
𝜏max = 16T/(πdᵣ³)
where:
- 𝜏max – Maximum nominal shear stress;
- T – Torque applied to the screw; and
- dᵣ – Minor diameter of the screw.
