Sled Ride Calculator

Snowy days are the best part of winter! Bring your children out in the snow to enjoy it (or enjoy it yourself 😉), but be safe and use our sled calculator to check if a slope is A-OK to sled on. Many injuries happen every year, mostly due to careless practices — that's why we're here to help!

Sledding, in particular, can be dangerous, but a little bit of science can help you decide if the hill you are looking at is safe or not. If you give us a couple of measurements 📏, our calculator will tell you:

• The maximum speed reached by your kid; and
• How far will they go after the slope has leveled out?

We are going to give you a couple of directions, but in the end, trust your judgment!

Why did we build this calculator? As scientists, we can't help but look at numbers — they are everywhere — and the statistics for sledding accidents are scary! 20,000 kids are injured every year! So we asked ourselves: How can we change those numbers with the help of numbers?

When you are done with sledding, or if you are looking for a more relaxing activity, why not build a snowman? We have the perfect tool for that: try our snowman calculator!

Did it snow tonight? Has the storm calmed a little? Brace yourself, wear thick gloves and warm clothes, and prepare to spend a day on the snow pulling sleds to the top of the slope. But maybe check that your kid is not projected into orbit at the end of their descent!

Sleds have always been one of the best ways to move on ice and snow! Think of skis, but without the instability and the uncomfortable boots. Also, they can be easily pulled: dogs, horses, and parents are the preferred choices for this.

Sledding is tons of fun, as everything regarding snow tends to be. But, like every activity involving some kind of action, it may be dangerous.

Let's be honest about it, without beating around the bush. Every year, around 20 thousand kids end up with some injuries due to sledding, in the US alone. It's a pretty huge number! Most of the injuries are fractures (and they are nasty to heal, trust us, especially if the kid loves the outdoors), and they mainly affect kids between the ages of 10 and 14. But following a couple of safety rules, you can help lower these figures, while keeping your kid (and yourself) safe!

Most of the injuries happen due to collisions, and speed is a crucial factor here! Follow the next steps to find out the speed of your kid during the descent.

It all boils down to an inclined plane problem — with friction!

When the sled slides down the slope, two forces act on it: gravity and friction. Let's analyze them:

• Gravity is defined as $F_g = m g$, where $m$ is the mass of the object (in this case, the sled plus the kid) times the gravitational acceleration $g = 9.81\ \text{m}/\text{s}^2$.
• Friction is the product of the normal force $F_N$ (the reaction to the weight of an object) times $\mu$, the friction coefficient: $F_f = \mu F_N$.

The force of gravity can be separated into two components, one perpendicular and one parallel to the slope's plane. They depend on the angle $\theta$ at which the plane is tilted. Here they are:

• The parallel component is equal to $F_{\Vert}=m g \sin{\left(\theta\right)}$. It's the only one controlling the speed of the object.
• Perpendicular component: $F_{⊥}=m g \cos{\left(\theta\right)}$. In a frictionless plane, this component would not matter at all, but in the presence of friction, it equals the normal force $F_N$.

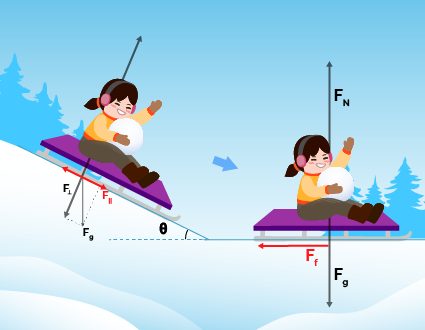
The acceleration of the system sled plus kid is given by the sum of the forces acting on it:

Now, we would like to introduce Isaac Newton, whose second principle states that force is equal to mass times acceleration, or $F = m a$.

Let's write the total force this way and look:

The mass appears on both sides of the equation, and we can cancel it out:

This is the acceleration! The fact that it doesn't depend on the mass is one thing us physicists will never get tired of — it's so counterintuitive, but Galileo didn't drop those weights for nothing!

The second part of the problem is located in the flat space after the slope has leveled out. In this part, the acceleration due to gravity is not present anymore, and only the friction acts on the sled. Now the normal force is not the perpendicular component anymore, but it takes all of the gravity force since $\theta = 0$ and $\sin{\left(\theta\right)} = 0$ and $\cos{\left(\theta\right)} = 1$. The friction is then $F_f = \mu m g$, and the total acceleration (which in this case is obviously a deceleration) is:

First, choose the type of sled you are using. This is extremely important! The type of material and the quality of the snow affect the ride a lot. For example, metal goes faster than every other common material used for sleds. Teflon (the polymer used in non-stick pans) is a runner-up, but it is not really common!

As for the snow, sledding on fresh, fluffy snow is not the best for a fast ride. Snow that is compact and a little wet makes for the fastest descents.

It's all a matter of friction: if you want to learn more about it, try our friction calculator.

You need to measure the slope you are going to use. It sounds difficult, but we swear, you won't need a measuring tape or anything else; you are going to be your own instrument.

1. Measure the slope's length. You can use any GPS app, or you can count your steps. To do this, determine your step length. Now, walk down the slope, counting your steps, and then multiply the number of steps you've just taken by your step length to obtain the slope's length. Easy peasy!
2. Measure the angle. This is a little bit more complicated. Check our graphics below, or use an app that allows you to simulate a protractor on your phone.

The motion of your kid on their sled is what physicists call uniformly accelerated motion. Lucky for us, there is a nice set of equations that can give you everything you need to know about the motion of an object with just a few initial values. Let's sketch them out!

We use these variables in the equations:

• $x$ — Position of the sled;
• $v$ — Velocity of the sled;
• $a$ — Acceleration of the sled;
• $t$ — Time at which we look at the sled; and
• $\ell$ — Length of the slope.

We call the initial position and velocity $x_0$ and $v_0$, respectively. In our calculations, we will assume both of these values to be $0$, as we're starting from rest on the top of the slope.

At any given time $t$, the speed of the sled is:

and its position is:

Now, to find the final speed down the slope, we just reverse the equation. We've already found the acceleration $a = g \sin{\left(\theta\right)} + \mu g \cos{\left(\theta\right)}$. Now we can find the time it takes to slide down the slope, $t_\text{slope}$. Ignoring the initial speed and position (as they're both $0$), we have:

Now it is possible to calculate the speed of the sled at the bottom of the slope:

The second part of the problem is again a uniformly accelerated motion. To be precise, we told you we should say "decelerated", but it all boils down to a minus sign!

The equations are the same, apart from the fact that now the initial speed $v_0$ equals the top speed at the end of the descent, i.e., $v_\text{bottom}$. From that value, and with the deceleration due to friction, we can easily find the length and the distance covered by the sled before it stops.

If you are a worried parent, put a helmet on your kid's head. But also remember that humans did without it for centuries. As for everything, just use common sense: don't let your child sled close to cars or trees.

It's a good idea to teach your kid some basic rules:

• Always ride feet first!
• Don't use the sled with more people than it's built for.
• If your kid wears a scarf, be sure everything is tucked in (why not a neck warmer then?).
• If possible, choose a steerable sled with brakes!
• Check what's after the slope: preferably nothing, and be careful to avoid the presence of roads and buildings.

The day is over — the sunset came and went, and it's already dark! We hope you had a safe and entertaining day on the snow, alone, with your kids or whoever you love. Now go get something warm — maybe in front of a fireplace!