Magnus Force Calculator

Our Magnus force calculator lets you calculate the total force produced by a spinning cylinder moving in a fluid medium. The Magnus effect phenomenon produces Magnus force that's caused due to the interaction between the moving fluid and the spinning object.

Are you curious to learn more about the Magnus effect? Read on to find answers to these questions and more:

• What is the Magnus effect, and how is the Magnus force generated?
• How to calculate the Magnus force generated by a spinning cylinder?
• How to use our Magnus force calculator to calculate the Magnus force?

Finally, you will find a numerical example demonstrating how to calculate the Magnus force using our calculator.

If you are a fan of ball-based games such as baseball, table tennis, or football, you might have noticed that spinning the ball creates a "magic" phenomenon where it deflects the ball from its original path. We call this "magic" phenomenon the Magnus effect. The word "Magnus" in the "Magnus effect" was named after the German physicist Gustav Magnus who studied the mysterious effect produced by spinning objects in the 19th century.

We define the Magnus effect as the phenomenon which produces a force on the spinning object moving in a fluid medium due to the generation of a pressure gradient across it.

In this particular case, our Magnus force calculator deals with the Magnus force produced by a spinning cylinder. The following explanation is, therefore only for the spinning cylinder case.

From the figure below, we can see the cross-section of a cylinder of length $\ell$ and radius $r$. The cylinder is spinning at a rate $f$ in a fluid medium of density $\rho$ which flows at the free stream velocity $v_\text{free}$.

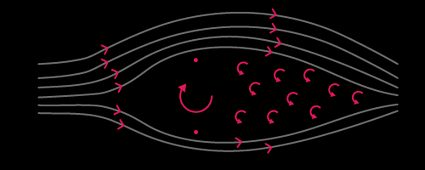
To understand the Magnus effect clearly, we can use the figure below.

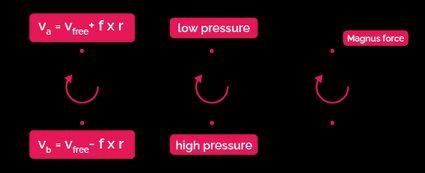
The figure above shows us how the Magnus effect is produced. We can follow the points below to understand the principle behind the generation of the Magnus effect:

1. Due to the spinning nature of the object, we have a difference in velocity between the top of the cylinder (indicated by point $a$) and the bottom (indicated by point $b$). We can observe this in the first part of the figure.
2. This velocity difference gives us a pressure difference between the top and the bottom (this follows from the good old Bernoulli's principle — refresh it at the Bernoulli equation calculator). We can see this in the second part of the figure.
3. In turn, the pressure gradient leads to the generation of a force called Magnus force pointing towards the lower pressure region. We can see this in the third part of the figure.

If the Magnus force is assisting to lift the object, we call it the Magnus lift force. Lift is an omnipresent force in aerodynamics: meet it at the lift coefficient calculator!

The Magnus force $L$ (a force and thus in $\text{N}$) is given by

where $G$ is the vortex strength in $\text{m}^2 / \text{s}$,

and $v_r$ is the cylinder's rotational speed in $\text{m} / \text{s}$,

In these equations, we also have the following symbols:

• As stated earlier, $r$ is the radius of the cylinder in $\text m$;
• $\ell$ is the length of the cylinder in $\text m$;
• $\rho$ is the density of the fluid in $\text{kg}/\text{m}^3$;
• $v_\text{free}$ is the free stream velocity of the fluid in $\text{m}/\text{s}$;
• $f$ is the rate at which the cylinder is rotating in $\text{rev}/\text{s}$.

We can also express the cylinder's rotational speed as the angular velocity, $\omega = 2\pi f$.

Combined, the equations above form what is known as the Kutta-Jowkowski equation:

Here's how to use our Magnus force calculator in a few simple steps:

• Input the cylinder's radius and length.
• Input the angular velocity or rotational velocity of the cylinder: you can learn how to find it using our angular velocity calculator.
• Determine the fluid density. You can either select the fluid medium from the drop-down list of fluids, or you can explicitly enter it in the density field.
• Finally, input the freestream velocity of the fluid.

With the above data, the calculator will provide you with the Magnus force $L$ that's generated by the Magnus effect.

You can find the acceleration due to the Magnus effect by calculating Newton's second law: this way you can quantify its effect on the trajectory of a projectile. Do this by modifying the calculated trajectory, adding the Magnus force in the proper direction, maybe alongside a modelization of the drag!

Let us see how to make use of our calculator to calculate Magnus force generated by a spinning cylinder. Consider the following values for various parameters involved:

• The cylinder has a radius of $r = 0.5\ \text{m}$ and length of $\ell = 10\ \text{m}$.
• The angular velocity of the cylinder is $\omega=20\ \text{rad}/\text{s}$.
• Fluid medium is air and its density is $\rho = 1.225\ \text{kg}/\text{m}^3$; and
• Fluid medium free stream velocity is $v_\text{free}=10\ \text{m}/\text{s}$.

Once we know these values, just insert them inside our calculator. Without any trouble, you will get the Magnus force produced by the cylinder: $3,\!848\ \text{N}$. (Psst! Use our special drop-down list to play around with different fluid mediums).

Awesome! Now you know how to use our calculator efficiently.