Triangle Proportionality Theorem Calculator

Q: What is a true statement about a 45-45-90 triangle?

A true statement about a 45-45-90 triangle is that the hypotenuse length is √2 times the length of either leg . Let's explain why 👇 From the sine definition: sin(45°) = opposite/hypotenuse hypotenuse = (1/sin(45°)) × opposite hypotenuse = √2 × opposite As the opposite sides are the legs, and both sides are equal: hypotenuse = √2 × leg

New

If you're studying geometry, a triangle proportionality theorem calculator like this one can be of great help.

Apart from knowing what the triangle proportionality theorem is, you also need to be familiar with how to use it. With our tool, you'll learn how to find the length of the lines that make up the segments intersected by DE (look at the image).

If you want to dig deeper, we present the triangle proportionality theorem proof in the FAQ section.

What is the triangle proportionality theorem?

In geometry, the triangle proportionality theorem says:

💡 If a line parallel to one side of a triangle intersects the other two sides, then the line divides those two sides proportionally.

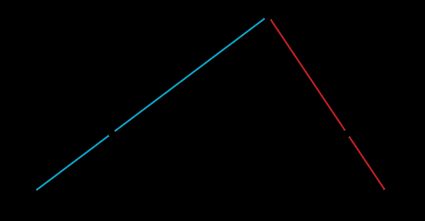
For example, for the triangle above, in which the line DE (parallel to the BC side) intersects the other two sides (AB and AC), the triangle proportionality theorem says:

\frac{\text{AB}}{\text{AD}} = \frac{\text{AC}}{\text{AE}}

You can draw lines across the three directions as long as they're parallel to one side, and the theorem will still be valid.

Now that you know the theory behind the calculation of the triangle proportionality theorem, you can look at other Omni tools similar to this triangle proportionality theorem calculator.

Other tools similar to this triangle proportionality theorem calculator

FAQs

What is a true statement about a 45-45-90 triangle?

A true statement about a 45-45-90 triangle is that the hypotenuse length is √2 times the length of either leg. Let's explain why 👇

From the sine definition:

sin(45°) = opposite/hypotenuse
hypotenuse = (1/sin(45°)) × opposite
hypotenuse = √2 × opposite

As the opposite sides are the legs, and both sides are equal:

hypotenuse = √2 × leg

What is the converse of the triangle proportionality theorem?

As for any theorem, we can state a converse for the triangle proportionality theorem:

If a line divides two sides of a triangle proportionally, that line must be parallel to the third side.

How do I prove the triangle proportionality theorem?

To prove the triangle proportionality theorem, consider a triangle ABC with a line DE parallel to BC (image below)

As parallel lines form congruent angles, then:

∠ADE ≅ ∠ABC and ∠AED ≅ ∠ACB
From the AA similarity criteria:

△ABC ~ △ADE
As the triangles are proportional:
AB/AD = AC/AE
Using the denominator subtraction property:

(AB - AD)/AD = (AC - AE)/AE
As AB - AD = DB and AC - AE = EC, we can achieve the proof of the triangle proportionality theorem:

DB/AD = EC/AE
That's how you prove the triangle proportionality theorem!