Last updated: Aug 05, 2024

Stress Concentration Factor Calculator

Q: What do you mean by stress concentration factor?

The stress concentration factor is the ratio of maximum stress to nominal stress at a location in an object under load. This parameter is useful to study the rise in stresses or discontinuity in the stress field due to the presence of geometric changes such as a hole, fillet, a chamfer, or even a change in cross-sectional area .

Q: How do I calculate stress concentration factor?

To calculate the stress concentration factor: Find the nominal stress at the point of interest. FInd the maximum stress for the material specimen. Divide the maximum stress by nominal stress to obtain the stress concentration factor, K t = σ_maximum / σ_nominal . Please note that the nominal stress calculation has specific formulae for different cases based on geometry and loading conditions.

Q: What do you mean by stress raisers?

The stress raises, or stress risers are the geometric locations where the stress is higher than the surrounding. In other words, the region where the localized stress distribution is higher than the stress in the specimen. Examples of stress raisers include fillets, chamfers, holes, weld joints, riveted or bolted joints, corners, and sharp edges.

New

Table of contents

What is stress concentration — Stress concentration factor Stress concentration factor equation Example: Using the stress concentration factor calculator FAQs

The stress concentration factor calculator is helpful to know about the increase in stresses in some of the regions of a machine part or a structure. The most basic example of this would be an infinitely long plate with a circular cutout. When the plate undergoes tension or compression or both, the stresses near the boundary of the cutout will be significantly higher and play an essential role in determining its failing circumstances.

Insights gained from this primary case could be valuable in designing and studying large machine parts that have holes for bolts and assemblies. One of the foremost uses of stress concentration is in composite structures where it plays a huge role due to the anisotropic nature of the material. To this end, the topic is of central interest in mechanics — solid mechanics, fatigue, and fracture mechanics. Read on to understand what stress concentration is and more.

What is stress concentration — Stress concentration factor

A structure or machine comprises several pieces of material — metals, composites, alloys, or polymers, joined together. Either permanently like welding or fastened with bolts or rivets. Some structures also have fillets or chamfers in the design to ensure certain functionality. These features in a structure are known as geometric discontinuities.

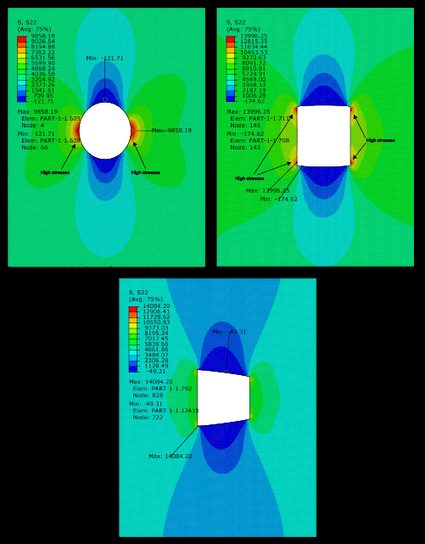
A geometric discontinuity could be a fillet, chamfer, a sharp edge, cracks, change in cross-sectional area (see cross sectional area calculator), a hole, or a cutout made on the structure. A higher value of stress is observed around these discontinuities when the structure is under load. Such localized higher stress values would contribute to microcracks and catastrophic material failure through cracks around the interface of discontinuities. Therefore, this parameter is of great interest for researchers in the field of fatigue and cycling loading and fracture mechanics.

The general equation for stress concentration is:

\small K_t = \frac{\sigma_{\rm max}}{\sigma_{\rm nom}}

Such that, the stress concentration factor, $K_t$ is the ratio of maximum (highest) stress ( $\sigma_{max}$ ) to the nominal stress ( $\sigma_{nom}$ ) of the gross cross-section.

Stress distribution in plates with cutouts; note the red regions having higher stress values.

Stress Calculators
Learn more about different type of stresses using our principal Stress Calculator, stress calculator, and hoop stress calculator.

Stress concentration factor equation

There are solutions for specific cases to estimate the stress concentration around a hole using analytical formulation. Such as the solution from E. Kirsch and Inglis for elastic stress around an elliptical hole in a plate. The maximum stress for an elliptical hole, having length and width as $a$ and $b$ in an infinitely long plate is:

\small \sigma_{\rm max} = \sigma_{\rm nom} \left ( 1 + 2 \frac{a}{b} \right )

For a circle, $a = b$ , therefore, the stress concentration factor is 3.

Similarly, for an anisotropic composite material, the stress concentration factor depends upon its elastic parameters such as moduli ( $E_x, E_y, G_{xy}$ ) and Poisson's ratio ( $\nu_{xy}$ ) which is given by the equation:

\footnotesize \begin{align*} K_t &= \frac{\sigma_{\rm max}}{\sigma_{\rm nom}} \\\\ &= 1 + \sqrt{2 \left [ \sqrt{\frac{E_x}{E_y}} - \nu_{xy}\right ] + \frac{E_x}{G_{xy}}} \end{align*}

Similarly, there are cases where solutions are available in various forms to predict the stress concentration factor analytically. In addition to this, there are methods to find the stress concentration factor experimentally. This method is applicable for especially determining the stress concentration around a hole. You can do it using optical techniques like photoelasticity and digital image correlation (DIC) systems. Such systems work on the principle that some plastic, polymers, and transparent materials exhibit fringe patterns related to the principal stress differences when under a source of illumination.

Due to advances in technology, this non-destructive optical technique is beneficial in studying strain and stress distribution for photoelastic specimens under elastic loads for both two and three-dimensional cases. In addition to experiments, you can also use any finite element (FE)-based simulation tool to study the stress concentration around geometric discontinuities. Once you know that the stress concentration factor is high, you can tweak your design using optimization techniques to reduce these values.

Example: Using the stress concentration factor calculator

Find the stress concentration for a plate with a square hole having nominal stress as 100 MPa. Take maximum stress as 150 MPa.

Select the mode of the calculator as ratio.
Enter the maximum stress as 150 MPa.
Fill in the nominal stress as 100 MPa.
Using the stress concentration factor formula:

\small \qquad K_t = \frac{\sigma_{\rm max}}{\sigma_{\rm nom}} = \frac{150}{100} = 1.5

Modes of calculator
You can also use the elliptical hole mode and the anisotropic composite mode to find the stress concentration factor for those specific cases.

FAQs

What do you mean by stress concentration factor?

The stress concentration factor is the ratio of maximum stress to nominal stress at a location in an object under load. This parameter is useful to study the rise in stresses or discontinuity in the stress field due to the presence of geometric changes such as a hole, fillet, a chamfer, or even a change in cross-sectional area.

How do I calculate stress concentration factor?

To calculate the stress concentration factor:

Find the nominal stress at the point of interest.
FInd the maximum stress for the material specimen.
Divide the maximum stress by nominal stress to obtain the stress concentration factor, K_t = σ_maximum / σ_nominal.

Please note that the nominal stress calculation has specific formulae for different cases based on geometry and loading conditions.

What is the stress concentration factor for an elliptical hole in an infinite plate?

The stress concentration factor for an elliptical hole in a plate is given by the equation:

K_t = σ_maximum = σ_nominal × (1 + 2 × (a / b))

where a and b are the major and minor axes, respectively. Now, in the case of a circular hole, this equation returns the answer as 3.

What do you mean by stress raisers?

The stress raises, or stress risers are the geometric locations where the stress is higher than the surrounding. In other words, the region where the localized stress distribution is higher than the stress in the specimen. Examples of stress raisers include fillets, chamfers, holes, weld joints, riveted or bolted joints, corners, and sharp edges.