Inductors in Parallel Calculator
Use the inductors in parallel calculator to determine the equivalent inductance in a parallel circuit. Continue reading to learn about parallel combination of inductors and the formula for calculating total inductance in a circuit. You will also find an example of how to calculate total inductance in a parallel circuit using the parallel inductance calculator.
If you want to calculate the equivalent inductance in a series circuit, you can check the inductors in series calculator.
Inductors in parallel
We can say that a group of coils (or inductors) are connected in parallel when one end of all the coils is connected to one point, and the other end of all the coils is connected to another point (see Figure 1). The AC source is connected across these two points.
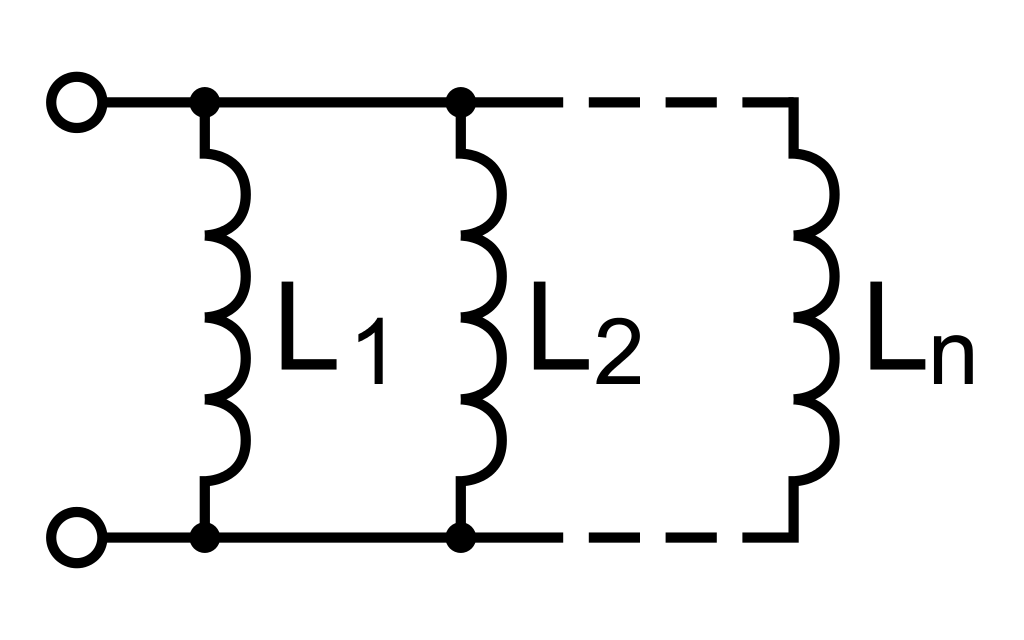
Formula for equivalent inductance in a parallel circuit
To determine the formula for the equivalent inductance when the inductors are connected in parallel, let us consider figure 1. If we connect an AC source across the combination, a self-induced e.m.f (electromagnetic field) appears in the coils due to the changing current .
Using the formula for self-inductance (or inductor formula) we can write:
In a parallel connection, the current gets divided as the inductors provide different paths through which it can flow. Hence, we can express the total current across the circuit as:
where, , , …, are the currents across the coils of inductance , , ..., .
Differentiating the above equation with respect to time, we get:
In a parallel connection, the voltage and the induced e.m.f (, , …, ) across each inductor is the same, i.e.:
Therefore, we can write:
The above equation gives the equivalent inductance of inductors in a parallel combination. We can also express the inductance in parallel formula as:
As you can see, the formula for a combination of inductors in parallel is similar to that for a combination of resistors in parallel. Explore more about them in our parallel resistor calculator
If you want to learn about the effective resistance offered by inductors, we recommend checking the inductive reactance calculator.
How to calculate total inductance in a parallel circuit
Let us see how to calculate the total inductance of a parallel circuit. Let Let , , and .
-
Using the equation for inductors in parallel, we get:
.
-
We will substitute the values of the inductors in the above equation:
-
Taking the reciprocal of the above, we can determine the equivalent inductance, i.e.:
How to use the inductors in parallel calculator
Now that we understand how to calculate the equivalent inductance in a parallel circuit, how about solving the same problem using our inductors in parallel calculator?
-
Using the drop-down menu, choose to Calculate... "equivalent inductance."
-
Enter the inductance values, i.e., , , and . You can add up to 10 inductors.
-
The inductors in parallel calculator will display the equivalent inductance, i.e., .
-
You can also use the parallel inductance calculator to find out the value of unknown inductance in a parallel circuit by changing the Calculate... option.
To calculate the self-inductance of a solenoid, visit our solenoid inductance calculator.
FAQs
How do I find the total inductance when inductors are connected in parallel?
To find the total inductance in a parallel circuit, proceed as follows:
- Add the reciprocal of individual inductances.
- Take the reciprocal of the value you get in step 1.
- Congrats! You have calculated the total inductance in a parallel circuit.
What happens when I connect two coils in parallel?
When we connect two coils of equal inductance in parallel, the effective inductance of the combination is reduced by a factor of half. The current through each inductor is also halved, but the voltage across each coil remains the same.
Do inductors have resistance?
An ideal inductor has no resistance. However, in actual practice, since the inductor coils are made from conductors, and all conductors have a finite resistance, inductors do have some resistance.
What is the inductance of three 10 mH inductors connected in parallel?
3.3 mH. According to the inductance in parallel formula, the reciprocal of the equivalent inductance of the three inductors connected in parallel is equal to the sum of the reciprocals of the individual inductances, i.e., 1/10 + 1/10 + 1/10 = 3/10. Hence, the inductance of three 10 mH inductors connected in parallel is 10/3 = 3.3 mH.
