Hyperfocal Distance Calculator

This hyperfocal distance calculator will help you find a camera's hyperfocal distance depending on its sensor size, focal length, and aperture area opening. In this calculator, you will learn:

• What hyperfocal distance is;
• How to find the hyperfocal distance of your camera; and
• The hyperfocal distance formula.

Learning about hyperfocal distance could help you improve your landscape photography game. Keep on reading to start learning 📸.

The prefix hyper itself seems to denote some extremity to the mentioned distance. And indeed it does.

Hyperfocal distance is the distance in which we can focus our camera to achieve the greatest or deepest depth of field. The hyperfocal distance depends on our chosen camera settings:

• Sensor size;
• Focal length; and
• Aperture area.

When focusing at the hyperfocal distance, our camera's depth of field will start at the hyperfocal near limit up to infinity. The hyperfocal near limit is the distance halfway towards the hyperfocal distance, as shown in the image below:

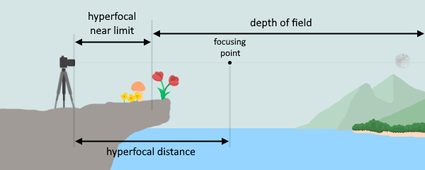
In other words, all the objects before the hyperfocal near limit will have a blurred image, and everything is "acceptably sharp" beyond that distance. However, since sharpness is subjective, we can say that the hyperfocal distance also varies depending on how much we consider something to be sharp. Factors affecting our perceived sharpness are visual acuity and the amount of enlargement we'll apply to our captured image when we make a printed copy.

Now that we know what hyperfocal is, how about we next discuss how to use our hyperfocal distance calculator? 🙂

Let's say we want to know the hyperfocal distance of a 35mm full-frame camera with a 50 mm lens and an aperture set at f/22. To use this tool to find the hyperfocal distance:

1. Firstly, we select 35mm full-frame from the sensor size list. If you have another camera with a different sensor size that's not on the list, select Custom sensor size from the options to enter its sensor width and height measurements.
2. Then, we enter 50 mm for the lens focal length.
3. Lastly, we pick f/22 from the aperture f-stop selection. This will display 22.627 for the focal ratio as additional information.

Upon doing these steps, our hyperfocal distance calculator will instantly display the hyperfocal near limit and the hyperfocal distance of our camera to be 1.94 m and 3.88 m, respectively.

Continuing our steps:

4. On the aperture f-stop options, we now select Custom. This option will clear the focal ratio and the hyperfocal distance values.
5. Click on the lock button 🔒 next to the focal ratio to show two more options: the lock toggle 🔒 and the autosave feature 💾 of our calculator. Click on the lock toggle to unlock the focal ratio. You'll see that we can now type values for the hyperfocal near limit and hyperfocal distance.
6. Enter 1.5 m for the hyperfocal near limit to discover our approximate focal ratio to now be 29.38. However, since we may not have a very specific focal ratio like that on our camera, we go to the next nearest aperture f-stop available to us.
7. Since our next available aperture f-stop is f/32, we select that to obtain our new hyperfocal near limit and hyperfocal distance with values of 1.38 m and 2.76 m, respectively. That will now cover the object 1.5 m away from us 🙂.

Of course, we don't forget that you may be wondering how to calculate hyperfocal distance yourself. That's why in the next section of this text, we'll discuss precisely that 🙂.

Learning how to calculate the hyperfocal distance is definitely another fun way to help you better understand what hyperfocal distance is. Calculating the hyperfocal distance takes only a few steps. Let's start with the hyperfocal distance formula, as shown below:

where:

• $H$ – Hyperfocal distance;
• $f$ – Focal length of lens used;
• $N$ – Aperture f-number; and
• $C$ – Circle of confusion limit.

Make sure that $f$ and $C$ are in millimeters to get a value for the hyperfocal distance in millimeters, too. In the formula above, we introduce the concept of the circle of confusion. We use the circle of confusion limit to determine the depth of field, and we find its value using this equation:

where:

• $d_{\text{av}}$ – Actual viewing distance of a printed photo version of an image;
• $d_{\text{sv}}$ – Standard viewing distance that a person can observe the said printed photo through a defined visual acuity;
• $\text{visual\ acuity}$ – Visual acuity at which an observer can tell the details in a printed photo at the standard viewing distance in terms of line pairs per mm (lp/mm);
• $\small{\text{diagonal}_\text{p}}$ – Diagonal measurement of the printed photo observed; and
• $\small{\text{diagonal}_\text{s}}$ – Diagonal measurement of the camera's sensor.

We can calculate the diagonals mentioned above with the help of the Pythagorean theorem as long as we know the widths and heights of our camera's sensor and the printed photo used in the visual acuity test.

Using the hyperfocal distance is perfect in taking landscape shots, cityscape pictures, and in astrophotography. However, since we're taking very deep depths of field and we're using small aperture sizes, capturing those photos in low-light situations would be difficult without the proper camera settings.

You can check out our shutter speed calculator to help you decide the duration of your shot. We also have our exposure calculator that you can use to check if your camera settings are perfect for any given lighting situation.

Hyperfocal near limit is the distance to the camera of the closest acceptably sharp object in a considered depth of field when focusing the camera at a point lying on the plane at the hyperfocal distance. We measure the hyperfocal near limit to be half the hyperfocal distance.

To calculate the hyperfocal distance:

1. First, we determine our camera's settings. Let's say we have a camera with 100 mm lens focal length (f), a circle of confusion (C) equal to 0.02884 mm, and an aperture f-stop of f/8, or a focal ratio (N) equal to 8.
2. Then, we define the hyperfocal distance formula as H = f + f²/(N×C).
3. Lastly, we substitute our known values to the formula to get: H = 0.1 + 0.1²/(8×0.02884) = 43,442.57 mm ≈ 43.44 m.

Use hyperfocal distance as your go-to focusing distance whenever you want to capture the entire scene while keeping nearby objects in focus. However, there are times when background objects can confuse the viewers about which object in your shot is the main subject. In those cases, it may not be best to use the hyperfocal distance and opt for a shallower depth of field.

Yes, sensor size affects the hyperfocal distance. The smaller the sensor size becomes, the farther the hyperfocal distance gets. However, as the hyperfocal distance moves away, so does the hyperfocal near limit — this decrease in sensor size results in more objects blurred in the foreground.

The hyperfocal distance we get using a 50 mm lens on a 35 mm full-frame camera at f/16 aperture is around 17.94 ft (or 5.47 m). Switching to an f/22 f-stop results in a closer hyperfocal distance of 12.73 ft (or 3.88 m). Decreasing the aperture size even more to f/32 will give us a hyperfocal distance of 9.05 ft (or 2.76 m).