Segment Area Calculator

Use this segment area calculator to quickly compute the area of a segment. It can also be used to find chord length and arc length. If you're unsure what a segment of a circle is or even what a chord of a circle is, don't feel embarrassed – just scroll down to find a few definitions and some self-explanatory images.

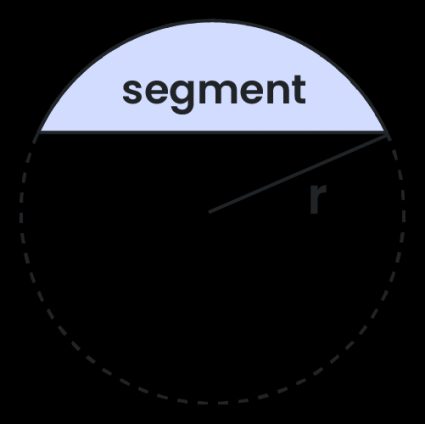
If you want to understand what the segment of a circle is, try to imagine cutting part of a circle off with a single, straight cut. And that's it! You've just created two parts of the circle, and the smaller one is called the circular segment. A more formal mathematical definition says that:

A circular segment is a region bounded by a chord and the arc of a circle (of less than 180°)

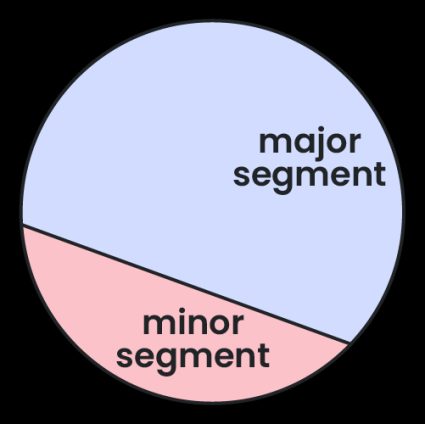
If it's equal to 180°, then it's simply a half circle – semicircle. According to some definitions, the central angle doesn't need to be smaller than 180° – in that case, you can say that cutting a circle with a line gives you two segments: a major segment and a minor segment.

Have a look at the picture below to help you visualize the difference between segment and sector, as those two names are sometimes confused:

A chord is a line that connects two points on a circle. The infinite line extension of a chord is called a secant. The special case of a chord is one that passes through the center of a circle – and that's the circle diameter, of course!

To find the circle segment area, you need to know at least two variables. In our segment area calculator, you'll find two popular formulas implemented:

1. Formula given radius and central angle

   A_segment = 0.5 × r² × (α – sin(α))

   Where does this formula come from? You can look at the segment area as the difference between the area of a sector and the area of an isosceles triangle formed by the two radii:

   A_segment = A_sector - A_triangle

   Knowing the sector area formula:

   A_sector = 0.5 × r² × α (learn more about this in the sector area calculator)

   An equation for the area of an isosceles triangle, given arm and angle:

   A_{isosceles triangle} = 0.5 × r² × sin(α) (learn more about this in the isosceles triangle area calculator).

   You can find the final equation for the segment of a circle area:

   A_segment = A_sector - A_{isosceles triangle} = (0.5 × r² × α) - (0.5 × r² × sin(α)) = 0.5 × r² × (α – sin(α))

2. Formula given radius and height

   A_segment = r² × arccos((r-h)/r) - (r-h) × √(2 × r × h - h²)

   where h is the height of a segment, also known as sagitta.

   This formula may be useful when you need to calculate, e.g., the volume of water in a pipe that is not completely full.

Let's find out how to use this segment area calculator. In our example, we want to find the area of the cross-section of a partially filled pipe:

1. Input the circle radius. Assume our pipe radius is 5 in.
2. Enter the second variable. Let's say that it's filled 3 inches high, so input that value into the height box.
3. There you go, that's it! Now we know that our segment area is equal to 19.8 in². Additionally, we determined the chord length (9.17 in), arc length (11.6 in), and central angle (132.84°).

Calculating the area of a segment is often needed in fields like engineering, architecture, and various forms of structural design.

You can calculate the segment area in three steps:

1. Determine the radius of the circle.

2. Calculate the central angle.

3. Apply the segment area formula:

   0.5 × r² × (α – sin(α))

Assuming the height is 2 cm, the segment area will be 11.18 cm². You can calculate it using this formula:

r² × arccos((r-h)/r) - (r-h) × √(2 × r × h - h²)

A minor segment is smaller than a semicircle, while a major segment is larger. The difference is based on whether the central angle is less or more than 180 degrees.